Part B. see the procedure
Part C. see the procedure
Step-by-step explanation:
we have
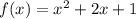 -----> equation A
-----> equation A
 -----> equation B
-----> equation B
Part B. Solve the system algebraically
equate the equation A and the equation B
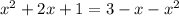
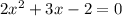
The formula to solve a quadratic equation of the form 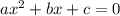 is equal to
is equal to
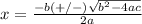
in this problem we have
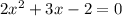
so
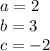
substitute in the formula
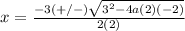
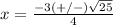
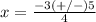

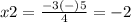
Find the values of y
For x=0.5
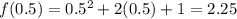
For x=-2
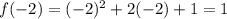
the solutions are the points
(0.5,2.25) and (-2,1)
Part C. Solve the system by graph
using a graphing tool
we know that
The solution of the non linear system is the intersection point both graphs
The intersection points are (0.5,2.25) and (-2,1)
therefore
The solutions are the points (0.5,2.25) and (-2,1)
see the attached figure
