Ok... so, we know that both pairs are conjugate of each other,
so.. both are complex solutions, namely, both are a+bi type
what's "a", we dunno, what is "b", we dunno either
but for z1, we know that a = m-2n-1 and b = 5m-4n-6
for z2, a = 3m-n-6 and b=m-5n-3
for those two folks to be conjugate of each other, they'd be
(a+bi)(a-bi)
notice, the "a"s are the same on z1 as well as z2
the "b" are the same also BUT the z2 "b" is negative,
in order to be a conjugate, regardless of that, the "b"s are the same
so, whatever (m-2n-1) is, is the same as (3m-n-6), since a = a
and whatever (5m-4n-6) is, will be the same value, since b =b,
the "a" and "b" in each conjugate, is the same value, thus one can say
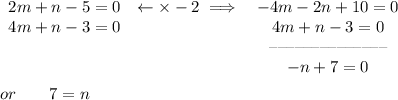
and pretty sure you can find "m" from there
once you have both, substitute in z1 or z2, to get the values for "a" and "b"
2
14 651
rating
![\begin{array}{lrcl}z_{1}/z_{2} & = & (0 - 4i)/(2 - 3i) &\\& =& -(4i)/(2 - 3i) \times (2 + 3i)/(2 + 3i)& \text{Multiply by conjugate}\\& =& -[4i(2 + 3i)]/[(2 - 3i)(2+ 3i)] &\text{Multply fractions} \\& =& -(8i - 12)/(4 + 9) &\text{Distribute and FOIL}\\& =& (12 - 8i)/13 &\text{Distribute and add}\\& =& \mathbf{12/13 - (8/13)i} & \text{Put into standard form}\\\end{array}\\\text{The quotient is }\boxed{\mathbf{12/13 - (8/13)i}}](/tpl/images/0173/9918/7e7d6.png)
Get help with homework
