a) Null hypothesis: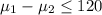
Alternative hypothesis: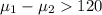
b) Calculate the statistic
We can replace in formula (1) the info given like this:
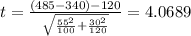
c) Critical value
The first step is calculate the degrees of freedom, on this case:
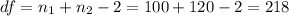
The significance level on this case is 0.05 or 5% so we need to find a quantile on the t distribution with 218 degrees of freedom that accumulates 0.95 of the area on the left and 0.05 of the area on the right tail and this value can be founded with the following excel code: "=T.INV(1-0.05,218)" and we got:

Conclusion
Since our calculated value is higher than the critical value we have enough evidence to reject the null hypothesis at 5% of significance and then we can conclude that the new product has a battery life more than two hours (120 minutes) longer than the leading product
Step-by-step explanation:
Data given and notation
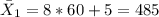 represent the mean for the new sample
represent the mean for the new sample
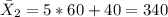 represent the mean for the old sample
represent the mean for the old sample
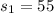 represent the sample standard deviation for the new sample
represent the sample standard deviation for the new sample
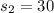 represent the sample standard deviation for the old sample
represent the sample standard deviation for the old sample
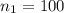 sample size selected for the new sample
sample size selected for the new sample
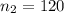 sample size selected for the old sample
sample size selected for the old sample
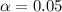 represent the significance level for the hypothesis test.
represent the significance level for the hypothesis test.
t would represent the statistic (variable of interest)
 represent the p value for the test (variable of interest)
represent the p value for the test (variable of interest)
a) State the null and alternative hypotheses.
We need to conduct a hypothesis in order to check if the new product has a battery life more than two hours longer than the leading product., the system of hypothesis would be:
Null hypothesis: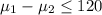
Alternative hypothesis: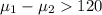
If we analyze the size for the samples both are higher than 30 but we don't know the population deviations so for this case is better apply a t test to compare means, and the statistic is given by:
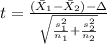 (1)
(1)
t-test: "Is used to compare group means. Is one of the most common tests and is used to determine whether the means of two groups are equal to each other".
b) Calculate the statistic
We can replace in formula (1) the info given like this:
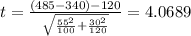
c) Critical value
The first step is calculate the degrees of freedom, on this case:
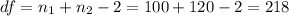
The significance level on this case is 0.05 or 5% so we need to find a quantile on the t distribution with 218 degrees of freedom that accumulates 0.95 of the area on the left and 0.05 of the area on the right tail and this value can be founded with the following excel code: "=T.INV(1-0.05,218)" and we got:

Conclusion
Since our calculated value is higher than the critical value we have enough evidence to reject the null hypothesis at 5% of significance and then we can conclude that the new product has a battery life more than two hours (120 minutes) longer than the leading product