The correct options are
(2) The vertex of the graph is (5, –37).
(3) The parabola has a minimum.
(4) The parabola opens up.
Step-by-step explanation: Given that the function  is written as follows in the vertex form :
is written as follows in the vertex form :

We are to select all the correct options.
We know that
for the STANDARD equation of a parabola, i.e., ![f(x)=a(x-h)^2+k,[\tex]</p<p<strongthe axis of symmetry is</strong [tex]x-h=0,](/tpl/images/1190/2302/e41f1.png)
the vertex of the parabola is (h, k),
the parabola has a minimum and opens up if a is positive, the parabola has a maximum and opens down if a is negative.
Comparing the equation (i) with the standard vertex form, we get
the axis of symmetry is given by
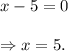
The vertex of the parabola will be
(h, k) = (5, -37).
Since the value of a is 10, positive, so the parabola has a minimum and opens up.
Thus, the correct options are
(2) The vertex of the graph is (5, –37).
(3) The parabola has a minimum.
(4) The parabola opens up.