QUESTION 1
The given function is
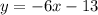
The domain of this function refers to all values of x for which y is defined.
The given function is defined for all real values of x.
The domain is all real numbers.
The correct answer is A
QUESTION 2
The equation in column 1 is
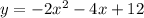
We obtain the vertex form as follows;
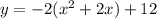

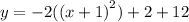
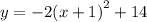
The x-value of the vertex is -1.
The equation in column 2 is

We can also find the x-value of the vertex using the formula,

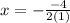
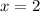
The correct answer is
B) The value found in column #1 is less than the value found in column #2
QUESTION 3
The height of the ball is modeled by
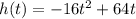
where t equals the time in seconds and h(t) represents the height of the ball at time t seconds.
The axis of symmetry can be found using the formula,
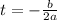
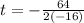

The correct answer is
A) t=2; it takes 2 seconds to reach maximum height and 2 seconds to fall back to the ground
QUESTION 4
The equation of axis of symmetry is given by the formula,

For the axis of symmetry of a given quadratic function to be zero, then the b-value of quadratic function should be zero.
The only equation from the given options whose b-value is zero is
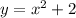
The axis of symmetry is
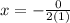

The correct answer is C