Mathematics : asked on anjoliej9

24.10.2020

X^2+8=10x complete the square

Step-by-step answer

09.07.2023, solved by verified expert

Sachin Kumar

m Master

Chemistry, Q&A Expert, I am a Assistant Professor in Maharaj Singh College Saharanpur, Uttar Pradesh, India

18 113

30 189 answers

4.8 rating

Unlock the full answer

The answer is

Step-by-step explanation:

X^2+8=10x complete the square, №18010170, 24.10.2020 09:27

It is was helpful?

Helpful Useless

Faq

Mathematics

X^2+8=10x complete the square

Step-by-step answer

P Answered by Master

The answer is

Step-by-step explanation:

Mathematics

Use completing the square to solve the equation x^2 - 10x = -8. which equation is used in the process? a. x^2 - 10x + 25 = 17 b. x^2 - 10x + 25 = -8 c. x^2 - 10x - 25 = -33

Step-by-step answer

P Answered by Specialist

Take 1/2 of the linear coefient and square it
add that to both sides

-10 is the linear coefient
1/2 of it is -5
squared it is 25
add 25 to both sides

x^2-10x+25=-8+25
x^2-10x+25=17
factor perfect square
(x-5)^2=17

answer is A

Mathematics

Use completing the square to solve the equation x^2 - 10x = -8. which equation is used in the process? a. x^2 - 10x + 25 = 17 b. x^2 - 10x + 25 = -8 c. x^2 - 10x - 25 = -33

Step-by-step answer

P Answered by Master

Mathematics

Follow the process of completing the square to solve x^2 - 10x + 8 = 0. what is the value of the constant that will be isolated on the right side of the equation in step 3? answer options are: a.-32 b.-12 c.-8

Step-by-step answer

P Answered by PhD

Start with x^2 - 10x + 8 = 0.

Take half of the coefficient of x; that is, take half of -10. Result: -5

Square this -5, and then add the resulting square to x^2 - 10x:

x^2 - 10 x + (-10/2) - (-10/2) = -8

Simplifying, x^2 - 10 x + 25 - 25 = -8

Rewriting: (x-5)^2 = 33

And so on.

The value of the const. that will be isolated on the rt. side of the equation in Step 3 is - 8.

Mathematics

Solving quadratic equations by completing the square explain! 1) r^2 - 4r - 91 = 7 2) x^2 - 10x + 26 = 8 3) k^2 - 4k + 1 = -5 4) b^2 + 2b = -20

Step-by-step answer

P Answered by Master

1. 2+sqrt(102)

2. 5+sqrt(5)

3. No Solution

4. No Solution

Step-by-step explanation:

1. r^2-4r+4-91=7+4 r^2-2r-2r+4 (r-2)^2-91=11 (r-2)^2=102 r-2=+sqrt(102)

1. x^2-10x+25+26=8+25 x^2-5x-5x+25 (x-5)^2+26=33 (x-5)^2=5 x-5=+sqrt(5)

1. k^2-4k+4+1=-5+4 k^2-2k-2k+4 (k-2)^2+1=-1 (r-2)^2=-2 No Solution

1. b^2+2b+1=-20+1 b^2+b+b+1 (b+1)^2=-19 No Solution

Mathematics

Follow the process of completing the square to solve x^2 - 10x + 8 = 0. What is the value of the constant that will be isolated on the right side of the equation in step 3? A.-8 B.-12 C.-32

Step-by-step answer

P Answered by Specialist

A. -8

Step-by-step explanation:

x^2-10x+8=0

then we subtract 8 from both sides

x^2-10x+8-8=0-8

x^2-10x=-8

The value on the right side is -8 in the process of completing the square.

Mathematics

Follow the process of completing the square to solve x^2 - 10x + 8 = 0. what is the value of the constant that will be isolated on the right side of the equation in step 3? -32 -12 -8

Step-by-step answer

P Answered by Specialist

To solve using completing square method we proceed as follows:
x^2-10x+8=0
x^2-10x=-8
but
c=(b/2)^2
c=(10/2)^2=25
thus we can add this in our expression to get
x^2-10x+25=8+25
factorizing the LHS we get:
(x-5)(x-5)=33
(x-5)^2=33
getting the square roots of both sides we have:
x-5=+/-√33
x=5+/-√33

Mathematics

Step-by-step answer

P Answered by PhD

Start with x^2 - 10x + 8 = 0.

Take half of the coefficient of x; that is, take half of -10. Result: -5

Square this -5, and then add the resulting square to x^2 - 10x:

x^2 - 10 x + (-10/2) - (-10/2) = -8

Simplifying, x^2 - 10 x + 25 - 25 = -8

Rewriting: (x-5)^2 = 33

And so on.

The value of the const. that will be isolated on the rt. side of the equation in Step 3 is - 8.

Mathematics

Solving quadratic equations by completing the square explain! 1) r^2 - 4r - 91 = 7 2) x^2 - 10x + 26 = 8 3) k^2 - 4k + 1 = -5 4) b^2 + 2b = -20

Step-by-step answer

P Answered by Master

1. 2+sqrt(102)

2. 5+sqrt(5)

3. No Solution

4. No Solution

Step-by-step explanation:

1. r^2-4r+4-91=7+4 r^2-2r-2r+4 (r-2)^2-91=11 (r-2)^2=102 r-2=+sqrt(102)

1. x^2-10x+25+26=8+25 x^2-5x-5x+25 (x-5)^2+26=33 (x-5)^2=5 x-5=+sqrt(5)

1. k^2-4k+4+1=-5+4 k^2-2k-2k+4 (k-2)^2+1=-1 (r-2)^2=-2 No Solution

1. b^2+2b+1=-20+1 b^2+b+b+1 (b+1)^2=-19 No Solution

Mathematics

Check if my answers are correct. will mark brainliest to whoever comments first! you, and god bless. 2. how is the graph of y equals -8x^2 - 2. different from the graph of y equals negative 8x squared.? (1 point) it is shifted 2 units to the left it is shifted 2 units to the right. *** it is shifted 2 units up. it is shifted 2 units down. a model rocket is launched from a roof into a large field. the path of the rocket can be modeled by the equation y equals negative 0.06 x squared plus 9.6 x plus 5.4 where x is the horizontal distance, in meters,

Step-by-step answer

P Answered by PhD

1. It is shifted 2 units down.

The graph of 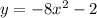y=-8x^2 -2 is shifted 2 units down with respect to the graph of 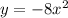y=-8x^2 . We can prove this by taking, for instance, x=0, and calculating the value of y in the two cases. In the first function:

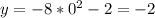
y=-8*0^2 -2=-2

In the second function:

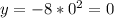
y=-8*0^2 =0

So, the first graph is shifted 2 units down.

2. 160.56 m

The path of the rocket is given by:

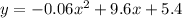
y=-0.06 x^2 +9.6 x +5.4

The problem asks us to find how far horizontally the rocket lands - this corresponds to find the value of x at which the height is zero: y=0. This means we have to solve the following equation

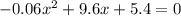
-0.06 x^2 +9.6 x+5.4 =0

Using the formula,

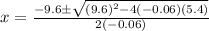
$x=\frac{-9.6 \pm \sqrt{(9.6)^2-4(-0.06)(5.4)}}{2(-0.06)}$

which has two solutions: 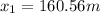x_1 = 160.56 m and x_2 = -0.56 m . The second solution is negative, so it has no physical meaning, therefore the correct answer is 160.56 m.

3. 27.43 m

The path of the rock is given by:

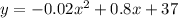
y=-0.02 x^2 +0.8 x +37

The problem asks us to find how far horizontally the rock lands - this corresponds to find the value of x at which the height is zero: y=0. This means we have to solve the following equation

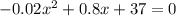
-0.02 x^2 +0.8 x+37 =0

Using the formula,

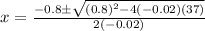
$x=\frac{-0.8 \pm \sqrt{(0.8)^2-4(-0.02)(37)}}{2(-0.02)}$

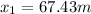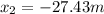which has two solutions: x_1 = 67.43 m and x_2 = -27.43 m . In this case, we have to choose the second solution (27.43 m), since the rock was thrown backward from the initial height of 37 m, so the negative solution corresponds to the backward direction.

4. (-2, 16) and (1, -2)

The system is:

y=x^2 -5x +2 (1)

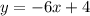y=-6x+4 (2)

We can equalize the two equations:

x^2 -5x+2 = -6x +4

which becomes:

x^2 + x -2 =0

Solving it with the formula, we find two solutions: x=-2 and x=1. Substituting both into eq.(2):

x=-2 --> y=-6 (-2) +4 = 12+4 = 16

x=1 --> y=-6 (1) +4 = -6+4 =-2

So, the solutions are (-2, 16) and (1, -2).

5. (-1, 1) and (7, 33)

The system is:

y=x^2 -2x -2 (1)

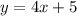y=4x+5 (2)

We can equalize the two equations:

x^2 -2x-2 = 4x +5

which becomes:

x^2 -6x -7 =0

Solving it with the formula, we find two solutions: x=7 and x=-1. Substituting both into eq.(2):

x=7 --> 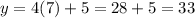y=4 (7) +5 = 28+5 = 33

x=-1 --> 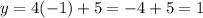y=4 (-1) +5 = -4+5 =1

So, the solutions are (-1, 1) and (7, 33).

6. 2.30 seconds

The height of the object is given by:

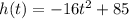
h(t)=-16 t^2 +85

The time at which the object hits the ground is the time t at which the height becomes zero: h(t)=0, therefore

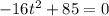
-16t^2 +85 =0

By solving it,

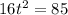
16t^2 = 85

$t^2 = \frac{85}{16}$

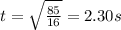
$t=\sqrt{\frac{85}{16}}=2.30 s$

7. Reaches a maximum height of 19.25 feet after 0.88 seconds.

The height of the ball is given by

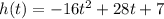
h(t)=-16t^2 + 28t + 7

The vertical velocity of the ball is equal to the derivative of the height:

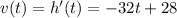
v(t)=h'(t)=-32t+28

The maximum height is reached when the vertical velocity becomes zero: v=0, therefore when

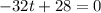
-32t + 28 =0

from which we find 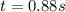t=0.88 s

And by substituting these value into h(t), we find the maximum height:

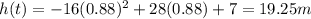
h(t)=-16(0.88)^2 + 28(0.88) + 7 = 19.25 m

8. Reaches a maximum height of 372.25 feet after 4.63 seconds.

The height of the boulder is given by

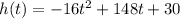
h(t)=-16t^2 + 148t + 30

The vertical velocity of the boulder is equal to the derivative of the height:

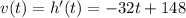
v(t)=h'(t)=-32t+148

The maximum height is reached when the vertical velocity becomes zero: v=0, therefore when

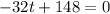
-32t + 148 =0

from which we find 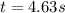t=4.63 s

And by substituting these value into h(t), we find the maximum height:

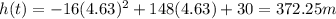
h(t)=-16(4.63)^2 + 148(4.63) + 30 = 372.25 m

9. 12 m

Let's call x the length of the side of the original garden. The side of the new garden has length (x+3), so its area is

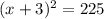
(x+3)^2 = 225

Solvign this equation, we find

$x+3 = \sqrt{225}=15$

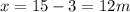
x=15-3=12 m

10. 225/4

In fact, if we write 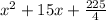$x^2 +15 x + \frac{225}{4}$ , we see this is equivalent to the perfect square:

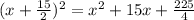
$(x+\frac{15}{2})^2 = x^2 +15 x +\frac{225}{4}$

11. -11.56, 1.56

The equation is:

x^2 +10 x -18 =0

By using the formula:

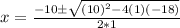
$x=\frac{-10 \pm \sqrt{(10)^2-4(1)(-18)}}{2*1}$

which has two solutions: x=-11.56 and 1.56.

12. -10.35, 1.35

The equation is:

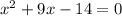
x^2 +9 x -14 =0

By using the formula:

$x=\frac{-9 \pm \sqrt{(9)^2-4(1)(-14)}}{2*1}$

which has two solutions: x=-10.35 and 1.35.

free Ask question

Today we have helped

1 110 students

just now

Mathematics

If (5^1/5)^5 = 25^x, then x = ?

s Answered by Specialist

just now

Mathematics

Clare’s aunt used a credit card to pay $42 for an outfit. Six weeks later, the credit card company charged her 2% interest. a. How much is the 2% interest?
b. How much will Clare’s aunt have to pay in total?

s Answered by Specialist

1 minute ago

Mathematics

20) Write a division number story with an answer of 1/4.

Be sure to ask a question.

p Answered by PhD

1 minute ago

Mathematics

on a certain Home Mortgage, there is a monthly payment of $9.43 for every $1,000 that is borrowed. What is the monthly payment on this type of loan if $135,000 is borrowed? NO LINKS!!!

s Answered by Specialist

1 minute ago

Mathematics

Find the mean of the distribution class 1-3 3-5 5-7 7-9. frequency 9 22 27 17

s Answered by Specialist

Get help with homework

X^2+8=10x complete the square

Step-by-step answer

Faq

Try asking the Studen AI a question.