Solve the following: 3! + 0! 2! * 1! A.) 3/2, №18010898, 02.05.2023 14:54

Mathematics

Solve the following: 3! + 0! 2! * 1! A.) 3/2 B.) 3 C.) 7/2

Step-by-step answer

P Answered by Master

3

Step-by-step explanation:

$\frac{3+0}{2\times1}$

$3+(\frac{0}{2})\times1$

$\mathrm{Divide}$

${0\div2}=0$

$3+0\times1$

3+0=3

$3\times1=3$

Lenvy~

Chemistry

In the quantum mechanical treatment of the hydrogen atom, which one of the following combinations of quantum numbers is not allowed? a.) n=1; l=3; ml=0 b.) n=3; l=1; ml=-1 c.) n=3; l=2; ml=-1 d.) n=3; l=3; ml=2 e.) n=3; l=2; ml=2

Step-by-step answer

P Answered by PhD

4

A) and d) are not allowed.

The quantum number l is restricted to be from 0 to n -1.

Then for a, where n = 1, l can only be 0

And for b., where n = 3, l can be 0, 1 and 2 (not 3).

Chemistry

In the quantum mechanical treatment of the hydrogen atom, which one of the following combinations of quantum numbers is not allowed? a.) n=1; l=3; ml=0 b.) n=3; l=1; ml=-1 c.) n=3; l=2; ml=-1 d.) n=3; l=3; ml=2 e.) n=3; l=2; ml=2

Step-by-step answer

P Answered by PhD

4

A) and d) are not allowed.

The quantum number l is restricted to be from 0 to n -1.

Then for a, where n = 1, l can only be 0

And for b., where n = 3, l can be 0, 1 and 2 (not 3).

StudenGPT

1. Use the table of values below to find the average rate of change over the interval . begin{tabular}{cc} & -3 & 0 -2 & -4 -1 & -6 0 & -6 1 & -4 hline & end{tabular} 4

Step-by-step answer

P Answered by Studen AI

The average rate of change is defined as the change in the y-values divided by the change in the x-values. From a mathematical perspective, it's analogous to the slope of the line that connects two points in a graph.

In the given problem, we have two x-values: -3 and 1. We need to find the corresponding y-values for each from the table provided.

From the table:
When x = -3, y = 0
When x = 1, y = -4

Now, let's calculate the average rate of change by substituting these values into the formula:

Average rate of change = (y2 - y1) / (x2 - x1)

Here, we're considering x = -3 as x1, x = 1 as x2, y = 0 as y1, and y = -4 as y2. Substituting these into the formula, we get:

Average rate of change = (-4 - 0) / (1 - (-3))

Simplifying this gives us:

Average rate of change = -4 / 4 = -1

So, the average rate of change over the interval [-3,1] is -1.

We will carefully follow all the notes you provided each time we solve for terms, ensuring no terms have been omitted, carefully cancelling out terms in fractions, paying close attention to signs, verifying our final answer, taking extra care when simplifying expressions, and ensuring accuracy in factoring expressions. In this calculation, those notes come in handy to make sure we have properly deal with the negative sign and correctly calculated the changes in the x and y values.

Mathematics

X=−2 x=−1 x=1 6(0.5x−1.5)+2x=−9−(x+6) x = − 2 – 6 ( 0.5 x − 1.5 ) + 2 x = − 9 − ( x + 6 ) x = − 1 – 6 ( 0.5 x − 1.5 ) + 2 x = − 9 − ( x + 6 ) x = 1 – 6 ( 0.5 x − 1.5 ) + 2 x = − 9 − ( x + 6 ) 4(0.5x−2)+3x=−14−(x+6) x = − 2 – 4 ( 0.5 x − 2 ) + 3 x = − 14 − ( x + 6 ) x = − 1 – 4 ( 0.5 x − 2 ) + 3 x = − 14 − ( x + 6 ) x = 1 – 4 ( 0.5 x − 2 ) + 3 x = − 14 − ( x + 6 ) 8(0.5x−3)+4x=−9−(x+6)

Step-by-step answer

P Answered by PhD

1

Given:

Three equations are

6(0.5x-1.5)+2x=-9-(x+6)

4(0.5x-2)+3x=-14-(x+6)

8(0.5x-3)+4x=-9-(x+6)

Three solutions are x=-2,x=-1,x=1 .

To find:

The solution of each equation from the given solutions.

Solution:

We have,

6(0.5x-1.5)+2x=-9-(x+6)

Using distributive property, we get

3x-9+2x=-9-x-6

5x-9=-15-x

Isolate variable terms.

5x+x=-15+9

6x=-6

Divide both sides by 6.

x=-1

Similarly,

4(0.5x-2)+3x=-14-(x+6)

2x-8+3x=-14-x-6

5x-8=-20-x

5x+x=-20+8

6x=-12

x=-2

Now,

8(0.5x-3)+4x=-9-(x+6)

4x-24+4x=-9-x-6

8x-24=-15-x

8x+x=-15+24

9x=9

x=1

Therefore, the solutions of given equations 6(0.5x-1.5)+2x=-9-(x+6) , 4(0.5x-2)+3x=-14-(x+6) and 8(0.5x-3)+4x=-9-(x+6) are x=-1, x=-2 and x=1 respectively.

Mathematics

X=−2 x=−1 x=1 6(0.5x−1.5)+2x=−9−(x+6) x = − 2 – 6 ( 0.5 x − 1.5 ) + 2 x = − 9 − ( x + 6 ) x = − 1 – 6 ( 0.5 x − 1.5 ) + 2 x = − 9 − ( x + 6 ) x = 1 – 6 ( 0.5 x − 1.5 ) + 2 x = − 9 − ( x + 6 ) 4(0.5x−2)+3x=−14−(x+6) x = − 2 – 4 ( 0.5 x − 2 ) + 3 x = − 14 − ( x + 6 ) x = − 1 – 4 ( 0.5 x − 2 ) + 3 x = − 14 − ( x + 6 ) x = 1 – 4 ( 0.5 x − 2 ) + 3 x = − 14 − ( x + 6 ) 8(0.5x−3)+4x=−9−(x+6)

Step-by-step answer

P Answered by PhD

1

Given:

Three equations are

6(0.5x-1.5)+2x=-9-(x+6)

4(0.5x-2)+3x=-14-(x+6)

8(0.5x-3)+4x=-9-(x+6)

Three solutions are x=-2,x=-1,x=1 .

To find:

The solution of each equation from the given solutions.

Solution:

We have,

6(0.5x-1.5)+2x=-9-(x+6)

Using distributive property, we get

3x-9+2x=-9-x-6

5x-9=-15-x

Isolate variable terms.

5x+x=-15+9

6x=-6

Divide both sides by 6.

x=-1

Similarly,

4(0.5x-2)+3x=-14-(x+6)

2x-8+3x=-14-x-6

5x-8=-20-x

5x+x=-20+8

6x=-12

x=-2

Now,

8(0.5x-3)+4x=-9-(x+6)

4x-24+4x=-9-x-6

8x-24=-15-x

8x+x=-15+24

9x=9

x=1

Therefore, the solutions of given equations 6(0.5x-1.5)+2x=-9-(x+6) , 4(0.5x-2)+3x=-14-(x+6) and 8(0.5x-3)+4x=-9-(x+6) are x=-1, x=-2 and x=1 respectively.

Mathematics

Please please help According to the probability distribution below, what is the expected value of X? X 0 1 2 3 4 5 P(X) 0.3 0.2 0.16 0.2 0.04 0.1 A.) The expected value is 0.1 B.) The expected value is 5 C.) The expected value is 2.5 D.) The expected value is 1.78

Step-by-step answer

P Answered by PhD

3

D.) The expected value is 1.78

Step-by-step explanation:

The expected value of a probability distribution is evaluated using the formula.

Expected Value, $E(X)=\sum^{n}_{i=1}x_iP(x_i)$

Therefore, from the given probability distribution, we have:

E(X)=(0*0.3)+(1*0.2)+(2*0.16)+(3*0.2)+(4*0.04)+(5*0.1)

E(X)=1.78

The Expected value of X is 1.78.

The correct option is D.

Mathematics

Which table shows a function that is decreasing only over the interval (–1, ∞)? A 2-column table with 6 rows. The first column is labeled x with entries negative 3, negative 2, negative 1, 0, 1, 2. The second column is labeled f of x with entries negative 1, negative 3, negative 5, negative 2, negative 1, 2. A 2-column table with 6 rows. The first column is labeled x with entries negative 3, negative 2, negative 1, 0, 1, 2. The second column is labeled f of x with entries negative 3, negative 5, negative 7, negative 6, 1, negative 1. A 2-column

Step-by-step answer

P Answered by Specialist

9

The correct answer is A (the first table)

Mathematics

A random sample of soil specimens was obtained, and the amount of organic matter (%) in the soil was determined for each specimen, resulting in the accompanying data. 1.10 5.09 1.20 3.50 0.69 4.47 0.97 1.59 4.60 5.02 4.67 5.22 3.31 1.17 0.76 0.32 0.55 1.45 0.14 4.47 2.69 3.98 3.17 3.03 2.21 1.17 1.57 2.62 1.66 2.05 The values of the sample mean and sample standard deviation are 2.481 and 1.616, respectively. Suppose we know the population distribution is normal, does this data suggest that the true average percentage of organic matter in such soil

Step-by-step answer

P Answered by Specialist

We conclude that the true average percentage of organic matter in such soil is different from 3%.

Step-by-step explanation:

We are given that the values of the sample mean and sample standard deviation are 2.481 and 1.616, respectively.

Suppose we know the population distribution is normal, we have to test the hypothesis that does this data suggest that the true average percentage of organic matter in such soil is something other than 3%.

Let 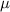$\mu$ = true average percentage of organic matter in such soil

SO, Null Hypothesis, 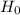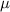H_0 : $\mu$ = 3% {means that the true average percentage of organic matter in such soil is equal to 3%}

Alternate Hypothesis, 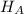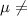H_A : $\mu \neq$ 3% {means that the true average percentage of organic matter in such soil is different than 3%}

The test statistics that will be used here is One-sample t test statistics because we don't know about the population standard deviation;

T.S. = 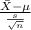$\frac{\bar X -\mu}{{\frac{s}{\sqrt{n} } } }$ ~ t_n_-_1

where, 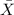$\bar X$ = sample mean amount of organic matter = 2.481%

s = sample standard deviation = 1.616%

n = sample of soil specimens = 30

So, test statistics = $\frac{0.02481-0.03}{{\frac{0.01616}{\sqrt{30} } } }$ ~ t_2_9

= -1.759

Now, P-value of the test statistics is given by;

P-value = P( t_2_9 > -1.759) = 0.046 or 4.6%

If the P-value of test statistics is more than the level of significance, then we will not reject our null hypothesis as it will not fall in the rejection region.If the P-value of test statistics is less than the level of significance, then we will reject our null hypothesis as it will fall in the rejection region.

Now, here the P-value is 0.046 which is clearly smaller than the level of significance of 0.05 (for two-tailed test), so we will reject our null hypothesis as it will fall in the rejection region.

Therefore, we conclude that the true average percentage of organic matter in such soil is different from 3%.