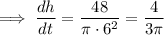PLEASE HELP THIS IS AP CALCULUS IM LOSING MY MIND A cup has the shape of a right circular cone. The height of the cup is 16 cm, and the radius of the
cm opening is 4 cm. Water is being poured into the cup at a constant rate of 3 cm. At what rate, in
cm per second, is the water level rising when the depth of the water in the cup is 6 cm? (The volume of a cone of height h and radius r is given by V=1/3pir^2h.

1. 1/64pi
2. 3/pi
3. 4/3pi
4. 9/4pi

3.  

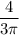
Step-by-step explanation:

Volume of a cone:

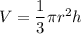
(where r is the radius and h is the height)

Given:

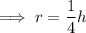
Therefore, substitute 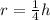into the volume formula to find the volume of the cone in terms of h:

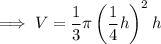
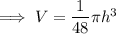
Differentiate with respect to h:

Volume is increasing at a constant rate of 3cm/s:

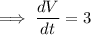
Use the chain rule to find

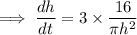
When h = 6:

SI=(P*R*T)/100

P=2000

R=1.5

T=6

SI=(2000*1.5*6)/100

=(2000*9)/100

=180

Neil will earn interest of 180

The solution is in the following image

y=2x+15

where y=Value of coin

x=Age in years

Value of coin after 19 years=2*19+15

=$53

Therefore, Value after 19 years=$53

F=ma

where F=force

m=mass

a=acceleration

Here,

F=4300

a=3.3m/s2

m=F/a

    =4300/3.3

    =1303.03kg

Here,

tip=18%of $32

tip=(18/100)*32

=0.18*32

=$5.76

Total payment=32+5.76=$37.76

Speed=Distance/time

Here,

distance=15m

time=1sec

speed=15/1=15m/sec

Distance=Speed*time

time=15min=15*60sec=900sec

Distance travelled in 15 min=15*900=13,500m

=13500/1000 km=13.5Km

The answer is in the image 

tip=18% of 75.45

     =18/100 * 75.45 = $13.581

Tip = $13.581