1) To write the equation in the standard form
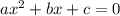
you need to take everything to the left side and multiply everything, if necessary, to get all whole integers:
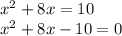
This will be your standard form of the equation.
2) To find a, b, c you just need to remember that:
- a is a coefficient in front of x^2
- b is a coefficient in front of x
- c is a constant with no x.
So, in your rewritten equation
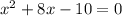
you have a = 1, b = 8, and c = -10
3) To solve the equation using quadratic formula, you need:
- find the Discriminant D, which is
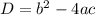
- if D < 0 there is no solution
- if D = 0 there is one solution
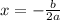
- if D > 0 there are two solutions which are
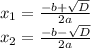
4) Let's solve the equation:
-
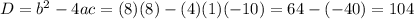
- 104 > 0 => there are 2 solutions
-
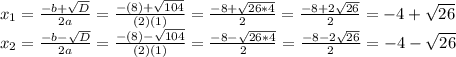
5) So, this is your solution. Good luck!