An object is dropped from a height of 150 feet. Its heights s at time, t is given by the equation s(t) =-16t^2+150 ,where s is measured in feet and t is measured in seconds.

Find the average rate of change of the height over the interval [1, 2.5).

O 1) -56 ft/sec

O2) 77 ft/sec

03) 56 ft/sec

O4) -35 ft/sec

The average rate of change of the height over the interval [1, 2.5] is - 56 feet per second.

Step-by-step explanation:

Let 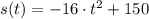. Geometrically speaking, average rate of change over a given interval (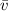), measured in feet per second, is determined by definition of secant line, which is defined:

(1)

Where:

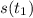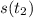, - Initial and final position of the object, measured in feet.

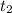, - Initial and final times, measured in seconds.

If we know that and 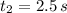, then the average rate of change over the interval :

The average rate of change of the height over the interval [1, 2.5] is - 56 feet per second.

The answer is in the image 

SI=(P*R*T)/100

P=2000

R=1.5

T=6

SI=(2000*1.5*6)/100

=(2000*9)/100

=180

Neil will earn interest of 180

The answer is in the image 

The total nom of  code that can be used is equal to 5+3 = 8

The answer is in the image 

The solution is in the following image

The answer is in the image 

Salesperson will make 6% of 1800

=(6/100)*1800

=108

Salesperson will make $108 in $1800 sales

The answer is in the image