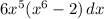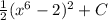
The indefinite integral can be found in more than one way. First use the substitution method to find the indefinite integral. Then find it without using substitution. Check that your answers are equivalent. 6x^5(x^6-2)dx

∫ =

Step-by-step explanation:

To find:

∫

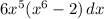
Solution:

Method of substitution:

Let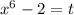

Differentiate both sides with respect to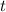

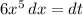
[use ]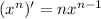

So,

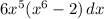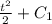∫ = ∫ = where 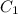is a variable.

(Use ∫ )

Put

∫ =

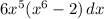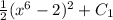
Use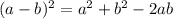

So,

∫ =

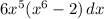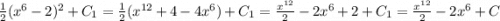
where

Without using substitution:

∫ = ∫ =

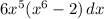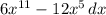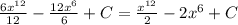
So, same answer is obtained in both the cases.

For 1 flavor there are 9 topping

Therefore, for 5 different flavors there will be 5*9 choices

No of choices= 5*9

=45 

The answer is in the image 

The answer is in the image 

y=2x+15

where y=Value of coin

x=Age in years

Value of coin after 19 years=2*19+15

=$53

Therefore, Value after 19 years=$53

The answer is in the image 

The solution is given in the image below

Here,

tip=18%of $32

tip=(18/100)*32

=0.18*32

=$5.76

Total payment=32+5.76=$37.76

The wood before starting =12 feet

Left wood=6 feet

Wood used till now=12-6=6 feet

Picture frame built till now= 6/(3/4)

=8 pieces

Therefore, till now 8 pieces have been made.

Salesperson will make 6% of 1800

=(6/100)*1800

=108

Salesperson will make $108 in $1800 sales