A fair coin is flipped three times. Let X be the number of heads observed. (a) Give both the possible values and probability mass function of X. (b) Find P(X ≥ 1) and P(X > 1). (c) Compute E[X] and V ar(X).

a)

The possible values are 0, 1, 2 and 3.

The probability mass function of X is:

P(X = 0) = 0.125

P(X = 1) = 0.375

P(X = 2) = 0.375

P(X = 3) = 0.125

b)

,

c) E(X) = 1.5, Var(X) = 0.75

Step-by-step explanation:

For it time the coin is flipped, there are only two possible outcomes. Either it comes up heads, or it comes up tails. Each toss is independent of other tosses. So we use the binomial probability distribution to solve this question.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

In which 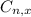is the number of different combinations of x objects from a set of n elements, given by the following formula.

And p is the probability of X happening.

The expected value of the binomial distribution is:

The variance of the binomial distribution is:

A fair coin is flipped three times.

This means that

Fair coin means that it is equally as likely to be heads or tails, so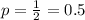

a) Give both the possible values and probability mass function of X.

The possible values are from 0 to n, so 0, 1, 2 and 3.

The probability mass function is the probability of each outcome. So

(b) Find P(X ≥ 1) and P(X > 1).

(c) Compute E[X] and Var(X).

For 1 flavor there are 9 topping

Therefore, for 5 different flavors there will be 5*9 choices

No of choices= 5*9

=45 

The answer is in the image 

The total nom of  code that can be used is equal to 5+3 = 8

The answer is in the image 

The solution is given in the image below

Speed=Distance/time

Here,

distance=15m

time=1sec

speed=15/1=15m/sec

Distance=Speed*time

time=15min=15*60sec=900sec

Distance travelled in 15 min=15*900=13,500m

=13500/1000 km=13.5Km

Salesperson will make 6% of 1800

=(6/100)*1800

=108

Salesperson will make $108 in $1800 sales

tip=18% of 75.45

     =18/100 * 75.45 = $13.581

Tip = $13.581

Area of rectangle= L*b

here,

L=12.4 cm

b= 8.8 cm

A= L*b

= 12.4 * 8.8 = 109.12 cm^2