Solve for x. 7x/−10=−42/5, №18009812, 19.01.2023 02:21

Mathematics

Solve for x. 7x/−10=−42/5

Step-by-step answer

P Answered by Specialist

x=12

Step-by-step explanation:

$-\frac{7x}{10} =-\frac{42}{5}$ multiply both sides by -10 as the first step to get x by itself

7x =84 . divide both sides by 7

x = 12

Mathematics

1. Solve for x. 4x + 12 = 60 2. Solve for x. 2x + 5 = 21 3. Solve for x. 4x - 7 = 5 4. Solve for x. 3b - 4 = 11 5. Solve for x. x3 = 33 6. Solve for x. ⅓ x + 6 = -8 7. Landon has 42 candies. He shares them with his friend Fred in a ratio of 3:5. How many candies does each person get? 8. Simplify the expression. 3x - 2 + 7 - 7x + 9 9. Simplify the expression. ⅓ (x - 3) + 12x 10. Jonah put in 12 colored tiles in a jar. There are 3 red tiles, 7 blue tiles, and 2 yellow tiles. What is the probability that he will select a red tile first, then select

Step-by-step answer

P Answered by PhD

1

Hello there!
1.)x=12 2.)x=8 3.)x=3 4.)x/b=5 5.)x=11 6.)x=-4 7.) about 25 8.)-4x+15 9.) 37/3 x +1 10.)63.74% chance 11.) 45 square feet 12.)26.66665

Hope this helps

Have a great day/night

Mathematics

1. Solve for x. 4x + 12 = 60 2. Solve for x. 2x + 5 = 21 3. Solve for x. 4x - 7 = 5 4. Solve for x. 3b - 4 = 11 5. Solve for x. x3 = 33 6. Solve for x. ⅓ x + 6 = -8 7. Landon has 42 candies. He shares them with his friend Fred in a ratio of 3:5. How many candies does each person get? 8. Simplify the expression. 3x - 2 + 7 - 7x + 9 9. Simplify the expression. ⅓ (x - 3) + 12x 10. Jonah put in 12 colored tiles in a jar. There are 3 red tiles, 7 blue tiles, and 2 yellow tiles. What is the probability that he will select a red tile first, then select

Step-by-step answer

P Answered by PhD

1

Hello there!
1.)x=12 2.)x=8 3.)x=3 4.)x/b=5 5.)x=11 6.)x=-4 7.) about 25 8.)-4x+15 9.) 37/3 x +1 10.)63.74% chance 11.) 45 square feet 12.)26.66665

Hope this helps

Have a great day/night

Mathematics

Triangle mkl is an isosceles triangle with mk congruent to ml. if mk=7x-15, kl=4x-5, and ml=10x-42, find x and the measure of each side

Step-by-step answer

P Answered by PhD

16

For given isosceles triangle ΔMKL with MK≅ML, x=9; MK=48, KL=31, ML=48.

Explanation:

An isosceles triangle has two of its 3 sides congruent.

For given ΔMKL, MK is congruent to ML (MK ≅ ML); i.e. length of side MK is equal to length of the side ML.

Given:

MK=7x-15

KL=4x-5

ML=10x-42

Equating MK with ML, we get:

MK=ML

7x-15 = 10x-42

Taking all x terms to the right,

42-15 = 10x-7x

27 = 3x

x=9

To find measure of each side MK, ML and KL; substitute the value of x=9 in corresponding equations.

MK = 7x-15

= (7×9) - 15

= 63-15

= 48

KL = 4x-5

= (4×9)-5

= 36-5

= 31

ML = 10x-42

= (10×9)-42

= 90-42

= 48

Therefore, for given ΔMKL with MK≅ML, x=9; MK=48, KL=31, ML=48.

Mathematics

Lesson 7 Extra Practice Equivalent Expressions Simplify each expression. 1. 5x + 3x 8x 2. 2x + x 3x 3. 6x + 8x 14x 4. 9x + 3x 12x 5. 7x + 4x 11x 6. 10x + x 11x 7. 3x + 2 + 4x 7x + 2 8. 15x + 3 + x 16x + 3 9. 2x + 5 + 6x 8x + 5 10. 7x + 2x + 4 9x + 42 11. 18x + 3x + 9 21x + 9 12. 6x + x + 8 7x + 8 Factor each expression. 13. 21x + 15y 3(7x + 5y) 14. 2x + 14y 2(x + 7y) 15. 15x + 10y 5(3x + 2y) 16. 12x + 18y 6(2x + 3y) 17. 10x + 30y 10(x + 3y) 18. 6x + 14y 2(3x + 7y) 19. 8x + 16y 8(x + 2y) 20. 45x + 27y 9(5x + 3y) 21. 54x + 48y

Step-by-step answer

P Answered by PhD

4

We need to solve all that?

I will need to solve that in a peace of paper okay because that is s long

Mathematics

Lesson 7 Extra Practice Equivalent Expressions Simplify each expression. 1. 5x + 3x 8x 2. 2x + x 3x 3. 6x + 8x 14x 4. 9x + 3x 12x 5. 7x + 4x 11x 6. 10x + x 11x 7. 3x + 2 + 4x 7x + 2 8. 15x + 3 + x 16x + 3 9. 2x + 5 + 6x 8x + 5 10. 7x + 2x + 4 9x + 42 11. 18x + 3x + 9 21x + 9 12. 6x + x + 8 7x + 8 Factor each expression. 13. 21x + 15y 3(7x + 5y) 14. 2x + 14y 2(x + 7y) 15. 15x + 10y 5(3x + 2y) 16. 12x + 18y 6(2x + 3y) 17. 10x + 30y 10(x + 3y) 18. 6x + 14y 2(3x + 7y) 19. 8x + 16y 8(x + 2y) 20. 45x + 27y 9(5x + 3y) 21. 54x + 48y

Step-by-step answer

P Answered by PhD

4

We need to solve all that?

I will need to solve that in a peace of paper okay because that is s long

Mathematics

1. A company produces two types of nutritional supplements; Energize and Excel. Energize contains 26 mg of vitamin A, 42 mg of vitamin C and 25 mg of an herbal supplement. Excel contains 68 mg of vitamin A, 61 mg of vitamin C and 15 mg of the herbal supplement. An athlete is told that he needs at least 489 mg of vitamin A, 1130 mg of vitamin C and 348 mg of the herbal supplement for optimal athletic performance. The athlete wants to take the supplements, but at the lowest possible cost. Energize pills cost 40 cents each, while Excel pills cost

Step-by-step answer

P Answered by PhD

1)A) Minimize C = 40x + 100y.

2) d) 7x + 8y ≤ 190, 5x + 4y ≤ 22, x ≥ 0, y ≥ 0.

3) B) Min C = 295x + 416y; s.t 23x + 15y ≥ 154, 17x + 25y ≥ 140, x ≥ 0, y ≥ 0.

Step-by-step explanation:

1) Energize pills cost 40 cents each, while Excel pills cost 100 cents each. If x represent the energize pills and y represent the excel pills, the objective function would be:

minimize cost = cost of energize pill + cost of excel pill

Minimize cost = 40x + 100y

2) Let x = the number of mountain bikes they produce, and let y = the number of racing bikes they produce.

7 hours of assemble time to produce a mountain bike and 8 hours of assemble time to produce a racing bike. Since the company has at most 190 hours for assemble time, it can be represented as:

7x + 8y≤ 190

5 hours of mechanical tuning to produce a mountain bike and 4 hours of mechanical tuning to produce a racing bike. Since the company has at most 22 hours for mechanical tuning, it can be represented as:

5x + 4y ≤ 22

Also assemble time and mechanical time must be greater than 0, hence:

x ≥ 0, y ≥ 0

3) The fieldwork constraint can be represented as:

23x + 15y ≥ 154

The time to be spent at university research center can be represented as:

17x + 25y ≥ 140

Both fieldwork time and time spent at university research center mut be greater than 0, hence:

x ≥ 0, y≥ 0

The cost equation is:

Minimize cost = 295x + 416y

Mathematics

1. A company produces two types of nutritional supplements; Energize and Excel. Energize contains 26 mg of vitamin A, 42 mg of vitamin C and 25 mg of an herbal supplement. Excel contains 68 mg of vitamin A, 61 mg of vitamin C and 15 mg of the herbal supplement. An athlete is told that he needs at least 489 mg of vitamin A, 1130 mg of vitamin C and 348 mg of the herbal supplement for optimal athletic performance. The athlete wants to take the supplements, but at the lowest possible cost. Energize pills cost 40 cents each, while Excel pills cost

Step-by-step answer

P Answered by PhD

1)A) Minimize C = 40x + 100y.

2) d) 7x + 8y ≤ 190, 5x + 4y ≤ 22, x ≥ 0, y ≥ 0.

3) B) Min C = 295x + 416y; s.t 23x + 15y ≥ 154, 17x + 25y ≥ 140, x ≥ 0, y ≥ 0.

Step-by-step explanation:

1) Energize pills cost 40 cents each, while Excel pills cost 100 cents each. If x represent the energize pills and y represent the excel pills, the objective function would be:

minimize cost = cost of energize pill + cost of excel pill

Minimize cost = 40x + 100y

2) Let x = the number of mountain bikes they produce, and let y = the number of racing bikes they produce.

7 hours of assemble time to produce a mountain bike and 8 hours of assemble time to produce a racing bike. Since the company has at most 190 hours for assemble time, it can be represented as:

7x + 8y≤ 190

5 hours of mechanical tuning to produce a mountain bike and 4 hours of mechanical tuning to produce a racing bike. Since the company has at most 22 hours for mechanical tuning, it can be represented as:

5x + 4y ≤ 22

Also assemble time and mechanical time must be greater than 0, hence:

x ≥ 0, y ≥ 0

3) The fieldwork constraint can be represented as:

23x + 15y ≥ 154

The time to be spent at university research center can be represented as:

17x + 25y ≥ 140

Both fieldwork time and time spent at university research center mut be greater than 0, hence:

x ≥ 0, y≥ 0

The cost equation is:

Minimize cost = 295x + 416y

Mathematics

1. solve this problem: –282 – (+1,017) = ? a. 735 b. –1,299 c. –735 d. 1,299 2. sue has a balance of $35 in her checking account. she writes one check for $10, and three checks for $9 each. what is her balance now? a. –$2 b. $54 c. –$11 d. $18 3. solve this inequality: 3b – 7 32 a. b 13 b. b 5 c. b 39 d. b 8.33 4. simplify and solve this equation: 4m + 9 + 5m – 12 = 42. a. m = –5 b. m = 4 c. m = -4 d. m = 5 5. simplify this expression: 13 + (–12) – (–5) = ? a. 30 b. –30 c. –6 d. 6 6. if four times a number plus 3 is 11, what is the number? a.

Step-by-step answer

P Answered by PhD

30

The correct answers are:

(1) (Option B) -1299

(2) (Option A) -2

(3) (Option A) b < 13

(4) (Option D) m = 5

(5) (Option D) 6

(6) (Option B) 2

(7) (Option A) p > 11

(8) (Option B) x = 6

(9) (Option A) h < –9

(10) (Option B) C = D ÷ AB

(11) (Option B) y = –45

(12) (Option A) y = 11

(13) (Option D) q = 13

(14) (Option A) M = K/LN

(15) (Option C) h = 63

(16) (Option C) –3p + 11

(17) (Option C) 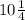$10\frac{1}{4}$

(18) (Option D) y = 31

(19) (Option D) R = E ÷ I

(20) (Option B) y = 4

Explanations:

(1) The Given Expression:

-282 - (+1017)

= -282 - 1017

= -1299 (Option B)

(2) The total balance = $35

One check = $10

Three checks = 3 * $9 = $27

Balance now = Total balance - One check + Three check = $35 - $10 - $27 = -2 (Option A)

(3) The given expression:

3b – 7 < 32

Add 7 on both sides:

3b -7 + 7 < 32 + 7

3b < 39

Divide by 3 on both sides:

$\frac{3b}{3}$

b < 13 (Option A)

(4) The given expression:

4m + 9 + 5m – 12 = 42

9m = 42 + 12 - 9

9m = 45

Divide both sides with 9:

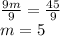
$\frac{9m}{9} =\frac{45}{9} \\ m=5$

The correct answer is m=5 (Option D)

(5) The given expression:

13 + (–12) – (–5)

= 13 - 12 + 5

= 6 (Option D)

(6) Mathematically, we can write "four times a number plus 3 is 11" as:

4x + 3 = 11

Where,

x = The number we require

4x = 11 - 3

4x = 8

x = 2 (Option B)

(7) The given expression:

12p + 7 > 139

Subtract 7 on both sides:

12p + 7 - 7 > 139 -7

12p > 132

Divide 12 on both sides:

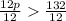
$\frac{12p}{12} \frac{132}{12}$

p > 11 (Option A)

(8) The given equation:

7x = 42

Divide the equation with 7 on both sides:

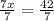
$\frac{7x}{7} =\frac{42}{7}$

x = 6 (Option B)

(9) The given expression:

9h + 2 < –79

Subtract 2 on both sides:

9h + 2 - 2 < -79 - 2

9h < -81

h < -9 (Option A)

(10) The given equation:

D = ABC

Now divide both sides with AB on both sides:

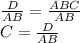
$\frac{D}{AB} =\frac{ABC}{AB} \\ C =\frac{D}{AB}$

Hence C = D ÷ AB (Option B)

(11) Given equation:

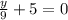
$\frac{y}{9} + 5 = 0$

Subtract 5 on both sides:

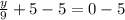
$\frac{y}{9} +5 -5 = 0 -5$

y = -5*9 = -45

y = -45 (Option B)

(12) Given equation:

12y = 132

Divide both sides by 12:

$\frac{12y}{12} =\frac{132}{12}$

y = 11 (Option A)

(13) The given equation:

3q + 5 + 2q – 5 = 65

5q = 65

Divide both sides with 5 and simplify:

q = 13 (Option D)

(14) Given formula:

K = LMN

To find M, divide both sides with LN:

$\frac{K}{LN} =\frac{LMN}{LN} \\ M = \frac{K}{LN}$

Hence the correct answer is 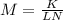$M = \frac{K}{LN}$ (Option A)

(15) Given formula:

h/9 = 7

Multiply both sides with 9:

h = 7 * 9

h = 63 (Option C)

(16) Given expression:

4p + 9 + (-7p) + 2

4p + 9 -7p +2

-3p + 11 (Option C)

(17) The given formula:

16y = 164

Divide both sides with 16:

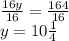
$\frac{16y}{16} =\frac{164}{16} \\ y = 10\frac{1}{4}$

Hence the correct answer is (Option C) 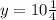$y = 10\frac{1}{4}$

(18) Given equation:

4y + 228 = 352

Subtract both sides with 228:

4y + 228 - 228 = 352 - 228

4y = 124

Divide both sides by 4 and simplify:

y = 31 (Option D)

(19) The given formula:

E = IR

To find R, divide both sides with I:

R = E ÷ I (Option D)

(20) Given equation:

6y - 20 = 2y - 4

=> 6y - 2y = -4 + 20

=> 4y = 16

Divide both sides with 4 and simplify:

y = 4 (Option B)

Mathematics

1.what is the simplified form of each expression? 7x^–8 × 6x^3 a. 42/x^5 b. 1/42x^5 c. 42x^11 d. 13x^–5 (–2x8) · 3y9 · 2x4 a. 3x^12y^9 b. –12x^72y^9 c. –12xy^21 d. –12x^y^9 2. find the simplified form of the expression. give your answer in scientific notation. (8 x 10^7) (7 x 10^4) a. 1.5 × 10^12 b. 5.6 × 10^12 c. 1.5 x 10^29 d. 5.6 x 10^29 (7 × 10^–4)(9 × 10^–10) a. 6.3 × 10^–13 b. 63 × 10^–13 c. 6.3 × 10^–15 d. 6.3 × 10^–16

Step-by-step answer

P Answered by PhD

3

Answer with explanation:

Ques 1)

A)

We are given a expression as:

$7x^{-8}\times 6x^3$

It could also be written as:

$(7\times 6)\times x^{-8}\times x^3\\\\\\=42\times x^{-8+3}\\\\\\=42x^{-5}\\\\\\=\dfrac{42}{x^5}$

Option: a is the answer.

B)

$(-2x^8)\times 3y^9\times 2x^4$

which is solved as follows:

$(-2\times 3\times 2)\times x^8\times y^9\times x^4\\\\\\=-12x^{8+4}\times y^9\\\\\\=-12x^{12}y^9$

Ques 2)

A)

The expression is:

$(8\times 10^7)(7\times 10^4)$

It is solved as:

$=(8\times 7)\times 10^7\times 10^4\\\\\\=56\times 10^{7+4}\\\\\\=56\times 10^{11}\\\\\\=5.6\times 10^{12}$

Option: b is the answer.

B)

$(7\times 10^{-4})(9\times 10^{-10})$

On simplifying:

$=7\times 9\times 10^{-4}\times 10^{-10}\\\\\\=63\times 10^{-4-10}\\\\\\=63\times 10^{-14}\\\\\\=6.3\times 10^{-14+1}\\\\\\=6.3\times 10^{-13}$

Option: a is the answer.

Solve for x.

7x/−10=−42/5

Step-by-step answer

Faq

Try asking the Studen AI a question.