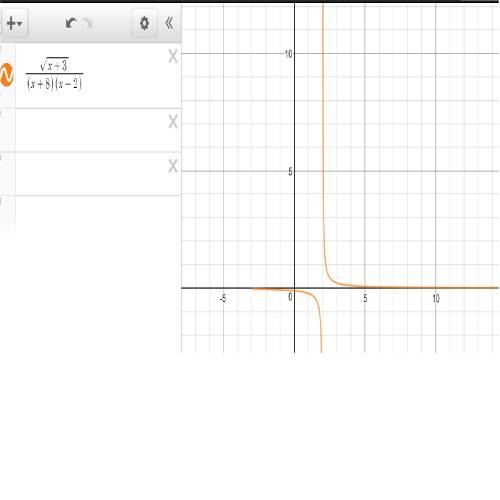
1) Find the domain of the given function. f(x) = square root of quantity x plus three divided by quantity x plus eight times quantity x minus two.
using a graphical tool see the attachment
the answer is C) x ≥ -3, x ≠ 2
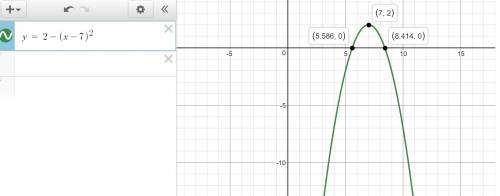
2. Identify intervals on which the function is increasing, decreasing, or constant.
g(x) = 2 - (x - 7)2
using a graphical tool
see the attachment
the answer is C) Increasing: x < 7; decreasing: x > 7
3. Perform the requested operation or operations.
f(x) = 4x + 7, g(x) = 3x2
Find (f + g)(x).
(f + g)(x) = f(x) + g(x)
(f + g)(x) = 4x + 7 + 3x^2
(f + g)(x) = 3x^2 + 4x + 7
The answer is C) 4x + 7 + 3x2
4. Perform the requested operation or operations.
f(x) = x minus five divided by eight. ; g(x) = 8x + 5, find g(f(x)).
f(x)=(x-5)/8 g(x)=8x+5
g(f(x))=8((x-5)/8)+5=x-5+5=x
the answer is B) g(f(x)) = x
5. Find f(x) and g(x) so that the function can be described as y = f(g(x)).y = nine divided by square root of quantity five x plus five.
y=f(g(x))=9/((5x+5) ^1/2)
let do
g(x)=5x+5...........so
f(x)= 9/( x^1/2)
the answer is A) f(x) = nine divided by square root of x. , g(x) = 5x + 5
6. A satellite camera takes a rectangular-shaped picture. The smallest region that can be photographed is a 4-km by 4-km rectangle. As the camera zooms out, the length l and width w of the rectangle increase at a rate of 3 km/sec. How long does it take for the area A to be at least 4 times its original size?
Original size- >4km*4km=16 km2
4 times its original size---------------4*(16km2)-----64 Km2----------- > 8 km by 8 Km
Therefore
3km----------------------------- 1 sec
(8km-4km)---------------------x
X=4/3=1.33 sec
The answer is D) 1.33 sec
7. Find the inverse of the function.
f(x) = the cube root of quantity x divided by seven. - 9
to solve, replace f(x) with y , switch x and y, solve for y and replace y with f⁻¹(x)
f(x)=((x/7)-9) ^(1/3)
replace f(x) with y
y=((x/7)-9) ^(1/3)
switch x and y
x=((y/7)-9) ^(1/3)
solve for y
x^3=((y/7)-9)
x^3+9=y/7
y=7(x^3+9)
the answer is C) f-1(x) = 7(x3 + 9)
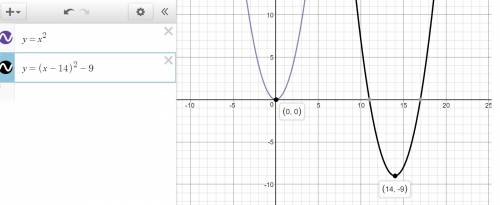
8. Describe how the graph of y= x2 can be transformed to the graph of the given equation.y = (x - 14)2 – 9
using a graphical tool see the attachment the answer is C) Shift the graph of y = x2 right 14 units and then down 9 units
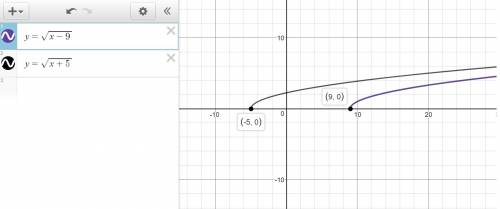
9. Describe how to transform the graph of f into the graph of g. f(x) = alt='square root of quantity x minus nine.' and g(x) = alt='square root of quantity x plus five. '
f(x)=(x-9) ^1/2 g(x)=(x+5) ^1/2
using a graphical tool see the attachment
the answer is C) Shift the graph of f left 14 units
10. If the following is a polynomial function, then state its degree and leading coefficient. If it is not, then state this fact.
f(x) = -16x5 - 7x4 – 6
The answer is B) Degree: 5; leading coefficient: -16
11. Write the quadratic function in vertex form.y = x2 + 4x + 7
Complete the square on the right side of the equation
Use the form ax2+bx+cax2+bx+c, to find the values of a, b, and c.
a=1,b=4,c=7
Consider the vertex form of a parabola.
a(x+d)2+e
Find the value of dd using the formula d=b/2a
d=4/(2*1)=2
Find the value of e using the formula e=c−b2/4a
e=7−4=3
Substitute the values of a, d, and e into the vertex form a(x+d)2+e
(x+2)2+3
The answer is A) y = (x + 2)2+ 3
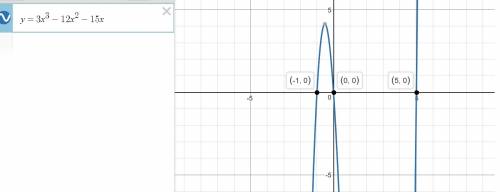
12. Find the zeros of the function.
f(x) = 3x3 - 12x2 - 15x
using a graphical tool (see the attachment)x1=-1
x2=0
x3=5
The answer is C) 0, -1, and 5
13. Find a cubic function with the given zeros.7, -3, 2
X1=7
X2=-3
X3=2
f(x)=(x-7)(x+3)(x-2)=(x2-4x-21)(x-2)=x3-6x2-13x+42
the answer is C) f(x) = x3 - 6x2 - 13x + 42
14. Find the remainder when f(x) is divided by (x - k).f(x) = 7x4 + 12x3 + 6x2 - 5x + 16; k = 3
f(x)=7(3)4+12(3)3+6(3)2-5(3)+16=946
The answer is the B) 946
15. Use the Rational Zeros Theorem to write a list of all potential rational zerosf(x) = x3 - 10x2 + 4x - 24
The constant term of () is -24
The leading
coefficient is 1.
We have to only consider the factors of the constant (leading
coefficient = 1)
The factors are 1, -1, 2, -2, 3, -3, 4, -4, 6, -6, 8,
-8, 12, -12, 24, -24
The answer is A) ±1, ±2, ±3, ±4, ±6, ±8, ±12, ±24