The time between arrivals of customers at an automatic teller machine is an exponential random variable with a mean of 4 minutes. Round yours answers to 4 decimal places. (a) What is the probability that more than three customers arrive in 10 minutes

0.7788 = 77.88% probability that more than three customers arrive in 10 minutes

Step-by-step explanation:

Exponential distribution:

The exponential probability distribution, with mean m, is described by the following equation:

In which is the decay parameter.

The probability that x is lower or equal to a is given by:

Which has the following solution:

The probability of finding a value higher than x is:

In this question, we have that:

The time between arrivals of customers at an automatic teller machine is an exponential random variable with a mean of 4 minutes, and we have three customers, which means that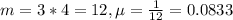

(a) What is the probability that more than three customers arrive in 10 minutes

This is P(X > 3). So

0.7788 = 77.88% probability that more than three customers arrive in 10 minutes

The answer is in the image 

SI=(P*R*T)/100

P=2000

R=1.5

T=6

SI=(2000*1.5*6)/100

=(2000*9)/100

=180

Neil will earn interest of 180

For 1 flavor there are 9 topping

Therefore, for 5 different flavors there will be 5*9 choices

No of choices= 5*9

=45 

The answer is in the image 

The answer is in the image 

For every 8 cars there are 7 trucks

Therefore,

Cars:Truck=8:7

Answer is B)8:7

y=2x+15

where y=Value of coin

x=Age in years

Value of coin after 19 years=2*19+15

=$53

Therefore, Value after 19 years=$53

F=ma

where F=force

m=mass

a=acceleration

Here,

F=4300

a=3.3m/s2

m=F/a

    =4300/3.3

    =1303.03kg

F=ma

where F=force

m=mass

a=acceleration

Here,

F=4300

a=3.3m/s2

m=F/a

    =4300/3.3

    =1303.03kg

Approximately it is aqual to 1300kg