Step-by-step explanation:
In any triangle, the sum of the lengths of any two sides should be strictly greater than the length of the third side. For example, if the length of the three sides are  ,
, 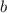 , and
, and  :
:
In this question, the length of the sides are  ,
, 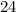 , and
, and  . The length of these sides should satisfty the following inequalities:
. The length of these sides should satisfty the following inequalities:
Since 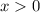 , the inequality
, the inequality 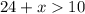 is guarenteed to be satisfied.
is guarenteed to be satisfied.
Simplify 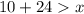 to obtain the inequality
to obtain the inequality 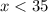 .
.
Similarly, simplify 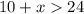 to obtain the inequality
to obtain the inequality  .
.
Since  needs to be a whole number, the greatest
needs to be a whole number, the greatest  that satisfies
that satisfies 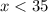 would be
would be 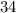 . Similarly, the least
. Similarly, the least  that satisfies
that satisfies  would be
would be 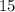 . Thus,
. Thus,  could be any whole number between
could be any whole number between  and
and 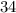 (inclusive.)
(inclusive.)
There are a total of 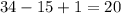 distinct whole numbers between
distinct whole numbers between 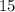 and
and 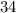 (inclusive.) Thus, the number of possible whole number values for
(inclusive.) Thus, the number of possible whole number values for  would be
would be 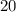 .
.